Автор: William E. Schiesser
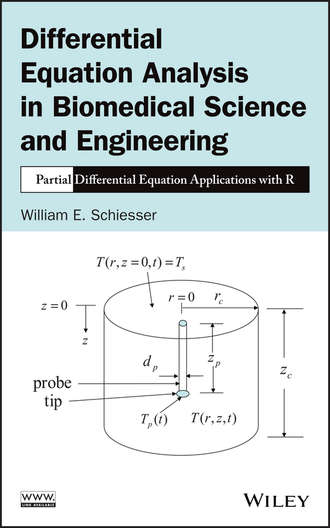
Differential Equation Analysis in Biomedical Science and Engineering
William E. Schiesser
Features a solid foundation of mathematical and computational tools to formulate and solve real-world PDE problems across various fields With a step-by-step approach to solving partial differential equations (PDEs), Differential Equation...
Spline Collocation Methods for Partial Differential Equations
William E. Schiesser
A comprehensive approach to numerical partial differential equations Spline Collocation Methods for Partial Differential Equations combines the collocation analysis of partial differential equations (PDEs) with the method of lines (MOL) in order...
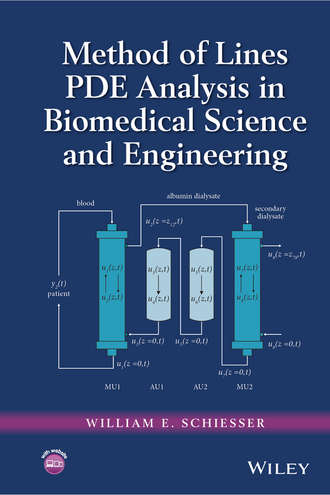
Method of Lines PDE Analysis in Biomedical Science and Engineering
William E. Schiesser
Presents the methodology and applications of ODE and PDE models within biomedical science and engineering With an emphasis on the method of lines (MOL) for partial differential equation (PDE) numerical integration, Method of Lines PDE Analysis...